Quantum Life Science

SUMMARY

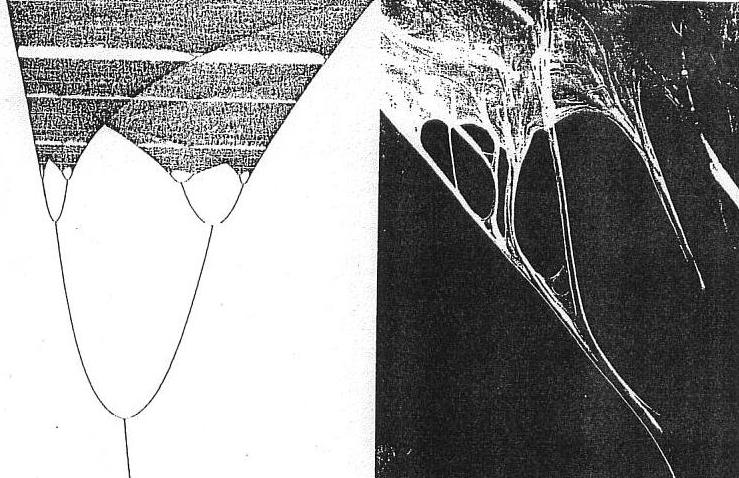
The 'Feigenbaum' as both mathematical and natural object

What Revolution ...?

In essence the rest of these pages seek to give some consideration to the science-wide revolution that has been wrought by mathematics through the emergence of chaos, fractals and complexity.  As will be seen from the next page, we can characterise this revolution as 'chaotic' under the imprimateur of the Royal Society here in the UK.

The primary spin-out has to be a change in science as we know it.  How big a change will only become apparent as the various disciplinary threads continue in their working until a point of resonance is realised, at which point there should be a full and free exchange of information, as is naturally required to realise the creation of a coherent vision. 

The new vision will be almost ineffably complex and on the way to its realisation will rewrite the history of science in all facets, but whilst undoing some of our old illusions, it will put in place a far more profound perspective on our place in the universe.

Three main outcomes have been introduced by the gedanken experiment:

1 

That the human genome can be understood as a 26 dimensional quantum system and the telomere as qubit.  Carbon, hydrogen and oxygen are the founding elements of the system which is then given over to the ordination and control of 23 free atom species significantly involved in cellular organisation.  This, the gedanken experiment implies, is achieved by means of five-system massive parallel processing. 
 
2 

That quantum theory - as proposed by Palmer (2008) - needs reformulation in the light of fractal geometry and chaos theory.  Although some of the first papers on quantum chaos can be found listed on ArXiv from as early as 1993, it seems that it is the realm of quantum theory that will be last to realise this revision - perhaps because of the very uncertain position of current quantum theory?  Palmer's proposal, indeed, arises directly out of a desire to use fractal geometry as a strategic means of resolving some of the continuing anomalies of current quantum theory, very much in the pragmatic mode of 'look at it another way'.

Larger scale or macro-physics has more readily employed this conceptual and metric tool and only two years after the advent of the Mandelbrot Set Chaotic Behaviour in General Relativity was the subject of a paper by John D. Barrow (1982, Physics Reports, v. 85: 01, May).  His closing paragraph to the conclusions of that analysis needs full rendition:

'We have shown that new ideas in non-linear dynamics [i.e., chaos theory] provide a natural description of the most general behaviour so far detected in Einstein's equations.  This enables the concept of a 'chaotic cosmology' to be made rigorous.  Finally, we should add, Einstein's equations create a new field of enquiry for dynamicists.  A field that should prove exceptionally fertile, for the unique self-interacting non-linearity of general relativistic systems hints at the presence of chaotic behaviour of unsuspected subtlety.'
(Italics and emphases are mine).

Allowing that we accept the premis of a fractal or chaotic universe, chaos in general relativity scales to the relative chaos of the quantum realm and by this we therefore expect chaos in quantum mechanics.

One expression of chaos in general relativity, we must suspect, lies in 'chaotic behaviour of unsuspected subtlety' where, indeed, the subtlety works to give clear trace of chaos as the underpinning to the evolution of man's own form. 

3
 
Mathematics itself seems now, as the 'real' subject of this scientific revolution, to need to complete the work begun by Leibniz in developing a natural mathematic based on scale invariant or transcendent operators in order to enable the identification and examination of recurrent patterns or systems at many scales of length and time.

A New Enlightenment

Is it possible, could it actually be the case that we really do have 'Nature's own geometry'?  This, of course, would be a realisation of the dream of ages, the universal mathematic by which then, naturally and easily enough, the unification of the sciences might then be far more reasonable and more readily tractable.  It was of course the dream of Descartes that gave us co-ordinate geometry, his key to the mathematical universe and one for which science remains eternally grateful.  

Fractal Geometry and Chaos theory together constitute what might be called 'The Mathematical Principles of Natural Philosophy', whereby these principles in combine are the complementary conceptual and mathematical tools by which we might understand all natural systems.  The Mandelbrot Set is our Rosetta Stone, iconographic of that realisation, whilst the Feigenbaum is iconographic signature to the founding dynamic of chaos.

Many do not accept the existence of such a mathematical reality, or perhaps the possibility of our possession of such a thing, yet it seems to be the case, as is ever being evidenced wherever fractality is found or applied, and this perception is now at least causing discussion in science and philosophy departments as to the possibility and meaning of realising our emergence within a fractal spacetime.  

Some would claim limit to the fractality, proposing phenomena not to be fractal if they do not meet their own measure, e.g., Ball (2009), proposes that 'fractal definition requires at least three orders of magnitude pattern repeat.'  A good scientist would be more interested in what has changed such that the expected pattern repeat is not immediately apparent. 

Others, more humbly, take instruction from what they find:

'The mathematicians are well acquainted with the difference between pure science, which has to do only with ideas, and the application of its laws to the use of life, in which they are constrained to submit to the imperfections of matter and the influence of accident.'  (Dr Johnson in the fourteenth 'Rambler', May 5, 1750).  

It is in the nature of the logic itself however, that if any part of the system is fractal, it is all fractal and such a simple principle works for this scientist at least.

Maths in the future - indeed, science in the future - must divide between the 'real sciences' and the 'classical', this latter being all that is not built in the natural mathematic.  Whilst that seems a simple bifurcation, it sets in train two others lines of enquiry - to understand how the classical and real might be related and for mathematics to effectively redefine itself in the production of a 'natural mathematic', as was the dream of Descartes and Leibniz, at least ...